How to Calculate the Distance to the Horizon

MediaScope

Editor

posted on 19 years ago — updated on 1 second ago

87
views

The distance to the horizon depends almost completely on the height above sea-level that the observer is looking from. Knowing this number is extremely useful and often necessary when navigating over the ocean or going on a hike, though just being curious is reason enough! There are also a few other factors that you might have to consider depending on where you are in the world and the time at which you are viewing, such as temperature and weather conditions. Once you have all the measures you need, you can quickly get the calculation and know the exact distance you are from the horizon.

Steps

Calculating Distance Using Geometry

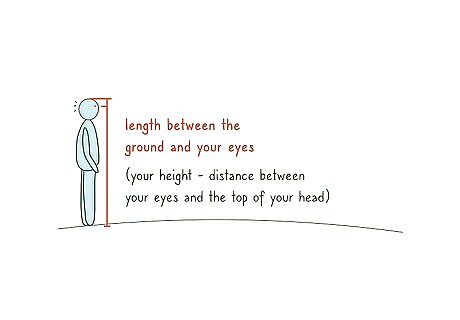
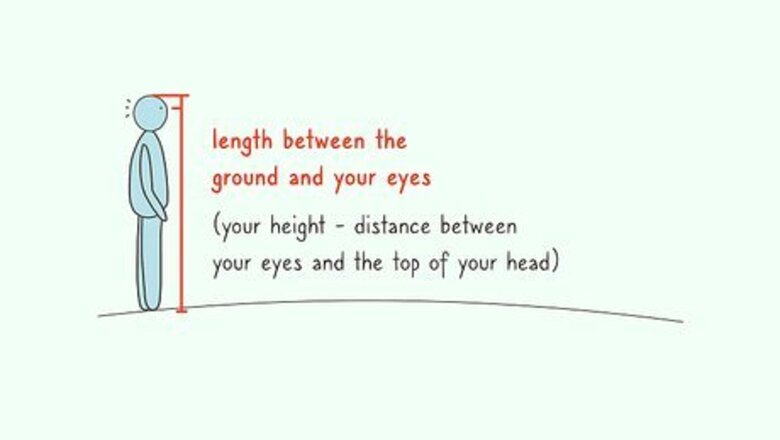
Measure your "height of eye." Measure the length between the ground and your eyes in meters or feet. One way to calculate this is to measure the distance between your eyes and the top of your head. Subtract this value from your total height and what will be left is the distance between your eyes and the surface you're standing on. If you are standing exactly at sea level, with the bottom of your feet level with the water, this is the only measurement you'll need.

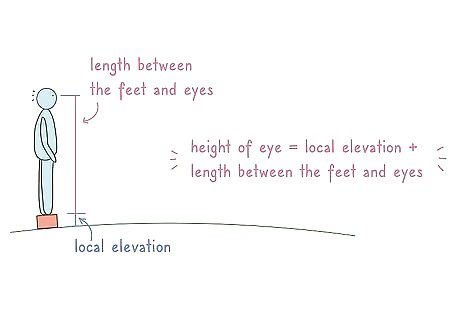
Add your "local elevation" if you're standing on a raised surface, such as a hill, building or boat. How many meters or feet above the true horizon are you standing? 1 meter? 4,000 feet? Add that number to your height of eye (in the same units, of course).

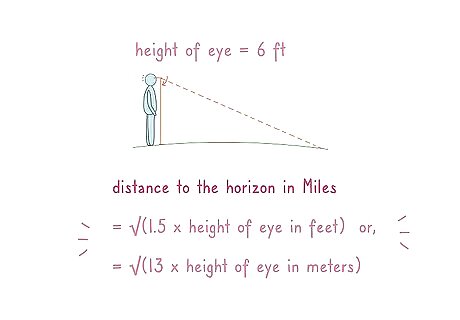
Multiply by 13m if you took the measurement in meters, or multiply by 1.5ft if you took the measurement in feet.

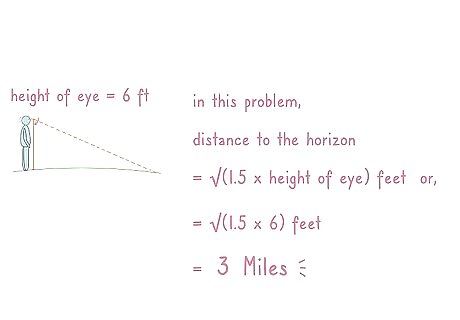
Take the square root to find the answer. If you used meters, your answer will be in kilometers, and if feet, the answer will be in miles. The distance calculated is a straight line from your eyes to the horizon. The actual distance you'll travel to get to the horizon will be longer because of surface curvature and (on land) irregularities. Proceed to the next method below for a more accurate (but complicated) formula.

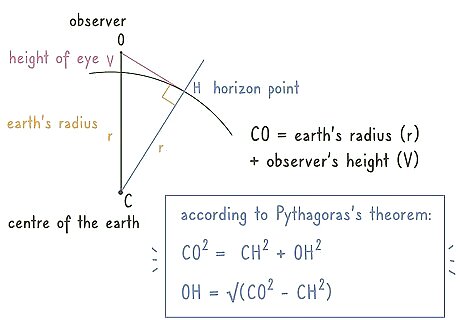
Understand how this calculation works. It's based on a triangle formed by your observation point (your eyes), the true horizon point (what you're looking at) and the center of the Earth. By knowing the radius of the Earth and measuring your height of eye and local elevation, that leaves only the distance between your eyes and the horizon as unknown. Since the sides of the triangle that meet at the horizon actually form a right angle, we can use the Pythagorean theorem (good old a + b = c) as the basis for this calculation, where:• a = R (the radius of the Earth)• b = the distance to the horizon, unknown• c = h (your height of eye) + R

Calculating Distance Using Trigonometry

Calculate the actual distance you'd have to traverse to get to the horizon by using the following formula. d = R * arccos(R/(R + h)), where• d = distance to horizon• R = radius of the Earth• h = height of eye

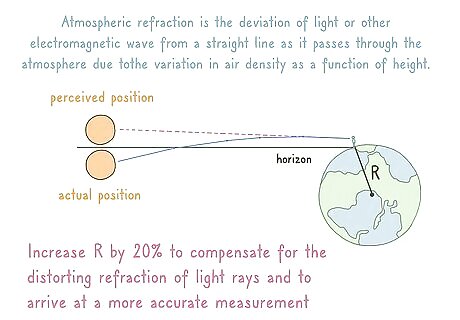
Increase R by 20% to compensate for the distorting refraction of light rays and to arrive at a more accurate measurement. The geometric horizon calculated using the method in this article may not be the same as the optical horizon, which is what your eye actually sees. Why is this? The atmosphere bends (refracts) light that is traveling horizontally. What this usually means is that a ray of light is able to slightly follow the curvature of the earth, so that the optical horizon is a bit further away than the geometric horizon. Unfortunately the refraction due to the atmosphere is neither constant nor predictable, as it depends on the change of temperature with height. There is therefore no simple way to add a correction to the formula for the geometric horizon, though one may achieve an "average" correction by assuming a radius for the earth that is a bit greater than the true radius.

Understand how this calculation works. This will calculate the length of the curved line that follows from your feet to the true horizon (shown in green in this image). Now, the arccos(R/(R+h)) portion refers to the angle that is made at the center of the Earth by the line going from the true horizon to the center and the line going from you to the center. With this angle, we multiply it by R to get the "arc length," which, in this case, is the distance that you are looking for.

Alternate Geometrical Calculation

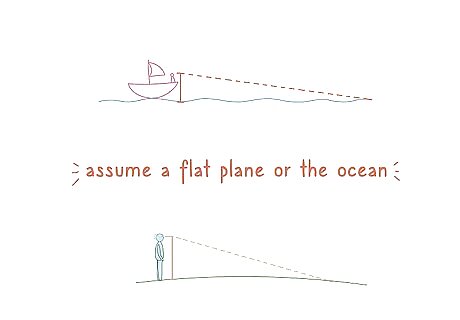
Assume a flat plane or the ocean. This method is a simpler version of the first set of instructions presented in this article, and applies only in feet and miles.

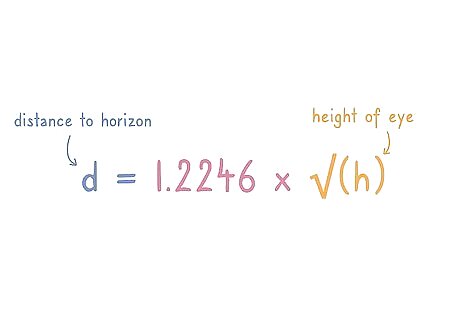
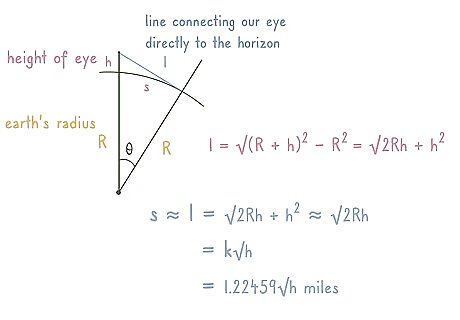
Solve for the distance in miles by plugging in your height of eye in feet (h) into the formula. The formula you will be using is d = 1.2246* SQRT(h).

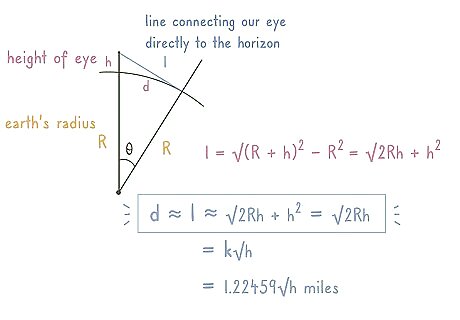
Derive the formula from the Pythagorean theorem. (R + h) = R + d. Solving for h (making the assumption that R>>h and expressing the radius of the earth in miles, approx. 3959) yields the expression:d = SQRT(2*R*h).