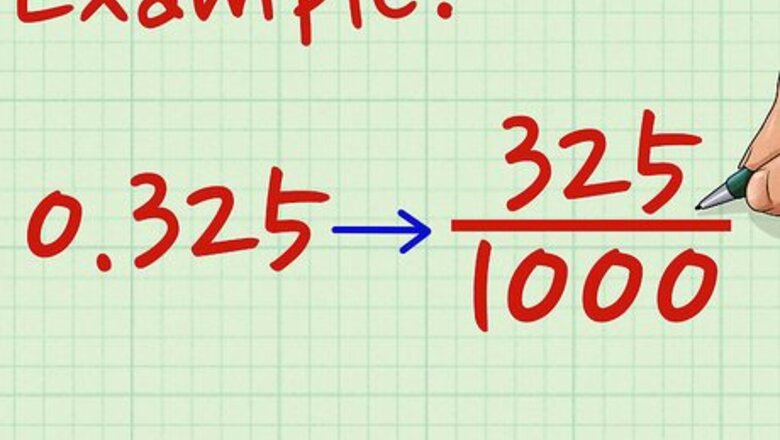
views
If the Decimal Terminates
Write down the decimal. If the decimal terminates, then it should end after one or several points after the decimal. Let's say you're working with the terminating decimal .325. Write it down.
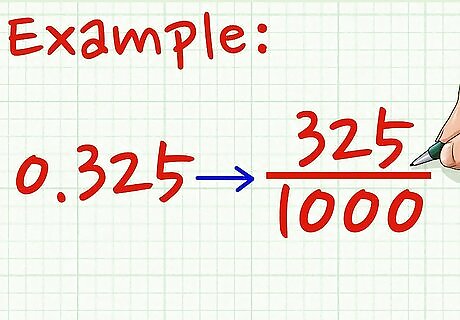
Convert the decimal to a fraction. To do this, count how many numbers there are after the decimal point. With the number .325, in this case, there are three numbers after the decimal point. So, put the number "325" over the number 1000, which is really the number 1 with three 0's after it. If you were working with the number .3, which is one number after the decimal points, then you could represent it as 3/10. You can also say the decimal aloud. In this case .325 = "325 thousandths." That sounds like a fraction! Write .325 = 325/1000.
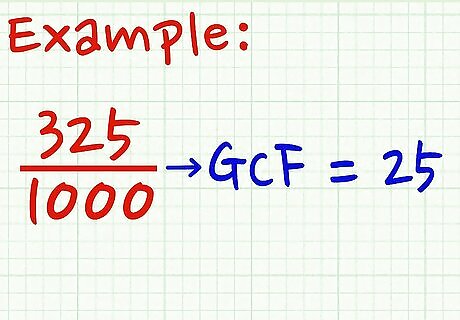
Find the greatest common factor (GCF) of the numerator and denominator of the new fraction. This is how you can simplify the fraction. Find the biggest number that divides evenly into both 325 and 1000. In this case, the GCF of both numbers is 25, because that's the largest number that goes evenly into both numbers. You don't have to look for the GCF right away. You can also use trial and error to simplify the fractions. For example, if you're working with two even numbers, keep dividing them by 2 until one of them becomes odd or you can't simplify further. If you're working with an even and odd number, try dividing them by 3. If you're working with numbers that end in a 0 or 5, divide them by 5.
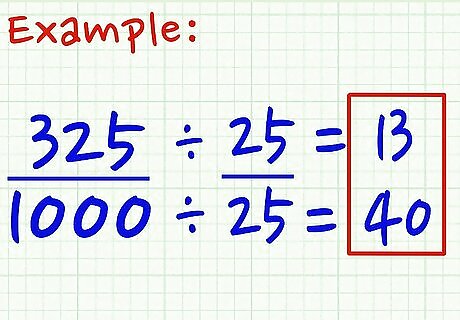
Divide both numbers by the GCF to simplify the fraction. Divide 325 by 25 to get 13 and divide 1000 by 25 to get 40. The simplified fraction is 13/40. So, .325 = 13/40. EXPERT TIP Joseph Meyer Joseph Meyer Math Teacher Joseph Meyer is a High School Math Teacher based in Pittsburgh, Pennsylvania. He is an educator at City Charter High School, where he has been teaching for over 7 years. Joseph is also the founder of Sandbox Math, an online learning community dedicated to helping students succeed in Algebra. His site is set apart by its focus on fostering genuine comprehension through step-by-step understanding (instead of just getting the correct final answer), enabling learners to identify and overcome misunderstandings and confidently take on any test they face. He received his MA in Physics from Case Western Reserve University and his BA in Physics from Baldwin Wallace University. Joseph Meyer Joseph Meyer Math Teacher To simplify fractions, you can divide both the numerator and denominator by a common factor. This creates a new, easier-to-use fraction with smaller components, but it represents the same value. For instance, if you divide both the numerator and denominator of 6/12 by 2, you get 3/6, which is equal to 1/2.
If the Decimal is Periodic
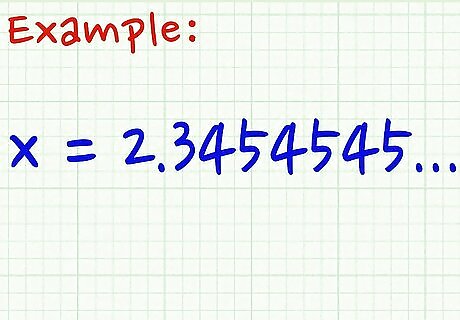
Write it down. A periodic decimal is a decimal with a repeating pattern that never ends. For example, 2.345454545 is a periodic decimal. This time, we'll be solving for x. Write x = 2.345454545.
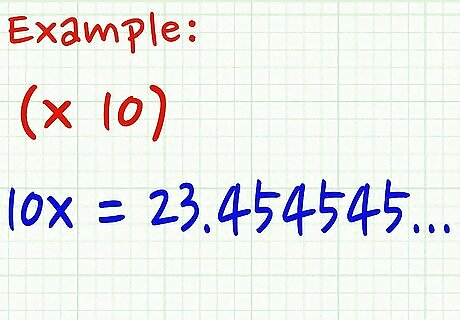
Multiply the number by a power of ten that would move any non-repeating part of the decimal to the left of the decimal point. In this example a single power of 10 will suffice, so write "10x = 23.45454545...." You have to do this because if you multiply the right side of the equation by 10, you have to multiply the left side of the equation by 10 too.

Multiply the equation by another power of 10 to move more numbers to the left of the decimal point. In this example, let's multiply the decimal by 1000. Write,"1000x = 2345.45454545...." You have to do this because if you multiply the right side of the equation by 1000, you have to multiply the left side of the equation by 1000 too.
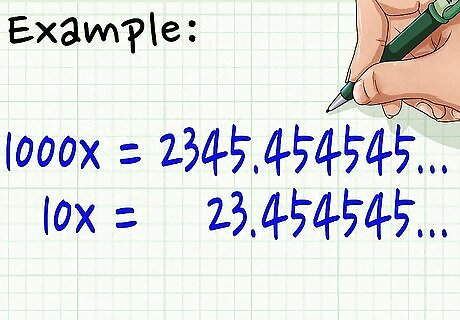
Place the variable and constant terms over each other. This will set them up to be subtracted. Now, place the second equation over the first, so that 1000x = 2345.45454545 is lined up over 10x = 23.45454545 just as it would be in a regular subtraction problem.
Subtract. Subtract 10x from 1000x to get 990x and subtract 23.45454545 from 2345.45454545 to get 2322. Now you have 990x = 2322.
Solve for x. Now that you have 990x = 2322, you can find "x" by dividing both sides by 990. So, x = 2322/990.
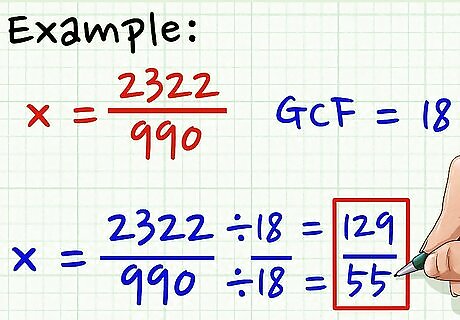
Simplify the fraction. Divide the numerator and denominator by any common factors. Compute the GCD of the numerator and denominator to ensure that you have fully simplified. In this example the GCD of 2322 and 990 is 18, so you can divide both 990 and 2322 by 18 to simplify the numerator and denominator of the fraction. 990/18 = 129 and 2322/18 = 129/55. Therefore, 2322/990 = 129/55. You're done.
















Comments
0 comment