views
Using a Number Line
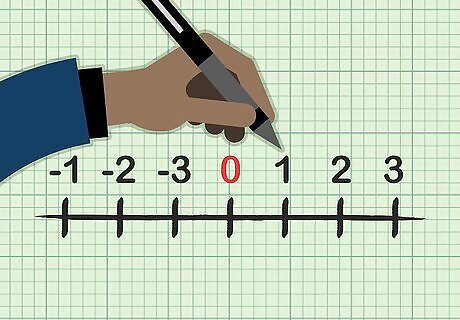
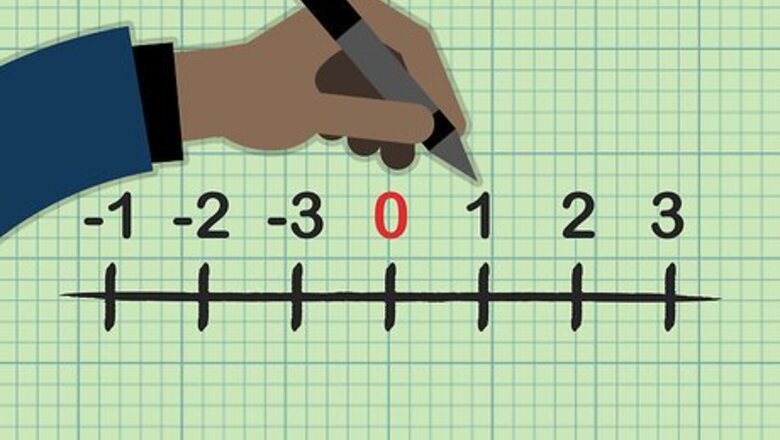
Draw your number line. Draw a long, horizontal line. Mark a short vertical line in the middle and label it "0." Make more marks to the right of 0 and label them 1, 2, 3, and so on in that order. Those are the positive numbers. Negative numbers go the opposite direction. Starting at 0 and moving left, draw more marks and label them -1, -2, -3, and so on.
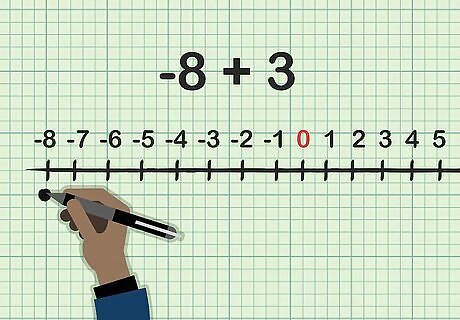
Start with the first number in your problem. Let's say you want to solve the problem -8 + 3. Find the first number, -8, on the number line. Draw a thick dot at that number.
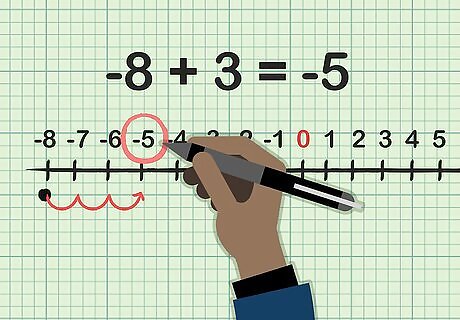
Review how to add positive numbers. On the number line, adding a positive number moves you to the right. For example, if you start at -8 and add 3, you move 3 marks to the right. The answer is where you end up: -5. This works no matter which number we start with.
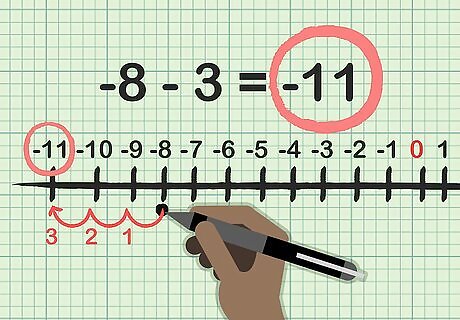
Subtract positive numbers by moving left. Subtracting a positive number moves you to the left of the number line. For example, you know that -8 - 3 = -11, because -11 is three marks left of -8.
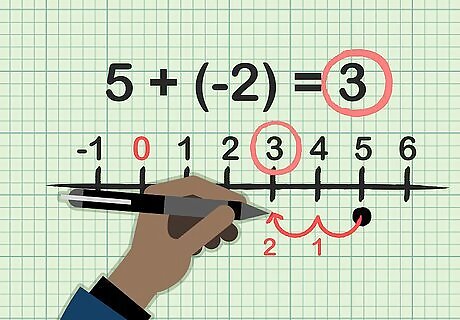
Add a negative number. Now let's try the other way around. This time, start at +5 on the number line and solve the problem 5 + (-2). Because the second number is negative, we change the direction we move on the number line. Adding normally moves to the right, but adding a negative number moves to the left instead. Start at +5, move 2 spaces to the left, and you end up at +3. So 5 + (-2) = 3. Here's another way to think of it: adding a negative number is the same as subtracting a positive number. 5 + (-2) = 5 - 2.
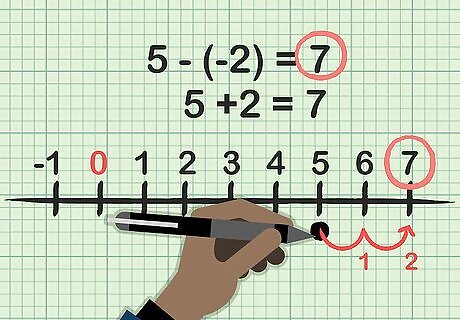
Subtract a negative number. Now try subtracting a negative number: 5 - (-2). Again, we're going to switch the normal direction, and move right instead of left. Start at +5, move two spaces to the right, and you end up at 7. Subtracting a negative number is the same as adding a positive number. 5 - (-2) = 5 + 2.
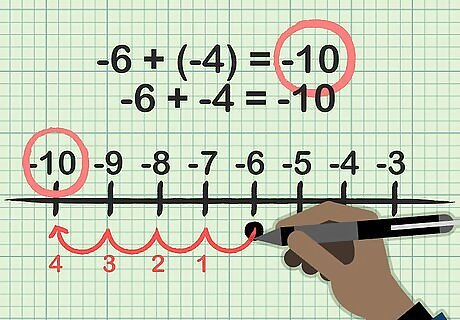
Add two negative numbers. Let's solve -6 + (-4). Start at -6 on the number line. Addition moves to the right, but the negative sign in front of the 4 changes our direction, so we move to the left instead. Move four spaces left of -6 and you'll land on -10, so -6 + (-4) = -10. Don't get confused by where you start on the number line. The first number only tells you where to begin on the number line. You'll always move right or left based on the type of problem and the second number.
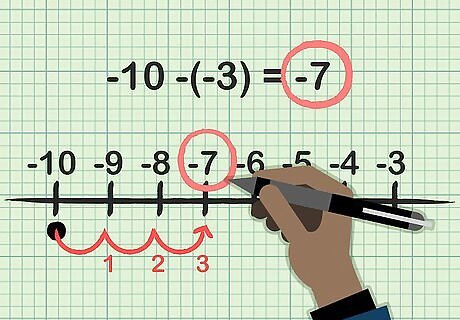
Subtract two negative numbers. Now let's solve -10 - (-3). Start at -10. Subtraction moves to the left but the negative in front of the 3 changes direction to the right instead. Move 3 places to the right and land on -3. The solution is -10 - (-3) = -7. Here's a memory aid: it takes two lines to draw the two negative signs. It also takes two lines to draw a plus sign, so - - is the same as +, moving to the right.
Without a Number Line
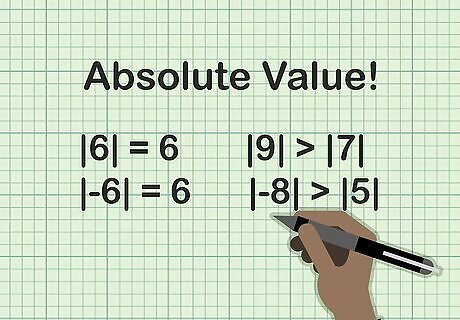
Learn about absolute value. For other problems, it's helpful to understand absolute value. The absolute value of a number is its distance from zero. The easiest way to find this is just to ignore the negative sign in front of it. Here are some examples: The absolute value of 6 is 6. The absolute value of -6 is also 6. 9 has a greater absolute value than 7. -8 has a greater absolute value than 5. It doesn't matter that one is negative.
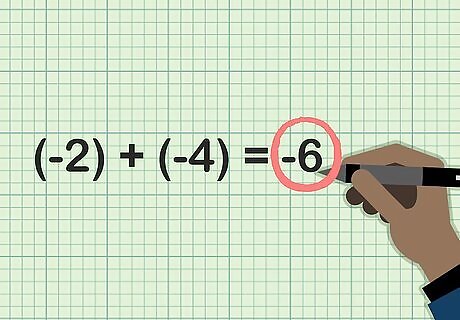
Add two negative numbers. Adding negative numbers together is just like adding positive numbers together, except that the answer has a "negative" sign in front of it. For instance, (-2) + (-4) = -6.
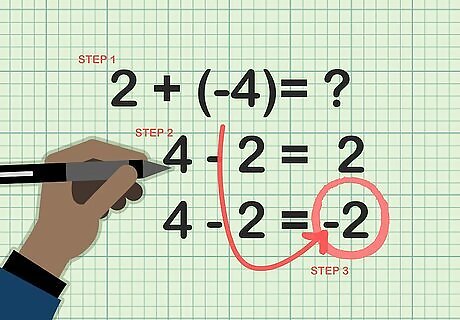
Add a positive and a negative number. For a problem like 2 + (-4), you might not know whether the answer will be positive or negative. If the number line doesn't help you figure it out, here's another way to solve it: Rearrange it so you're subtracting the smaller absolute value from the larger one. Ignore the negative sign for now. For our example, write 4 - 2 instead. Solve that problem: 4 - 2 = 2. This isn't the answer yet! Look at the original problem and check the sign (+ or -) of the number with the largest absolute value number. 4 has a higher value than 2, so we look at that in the problem 2 + (-4). There's a negative sign in front of the 4, so our final answer will also have a negative sign. The answer is -2.
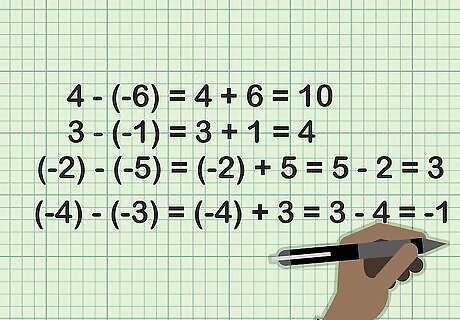
Subtract a negative number. Subtracting the negative is the same as adding a positive. For instance, 4 - (-6) = 4 + 6. This gets a little harder when you start with a negative number as well. Once it's an addition problem, you can switch the order of the two numbers and turn it into an ordinary subtraction problem. Here are a few examples: 3 - (-1) = 3 + 1 = 4 (-2) - (-5) = (-2) + 5 = 5 - 2 = 3 (-4) - (-3) = (-4) + 3 = 3 - 4 = -1
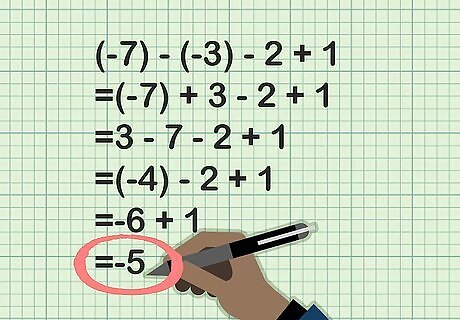
Solve problems with more than two numbers. If you have a long chain of numbers, just use these steps to solve them two at a time. Here's an example: (-7) - (-3) - 2 + 1 =(-7) + 3 - 2 + 1 =3 - 7 - 2 + 1 =(-4) - 2 + 1 =-6 + 1 =-5



















Comments
0 comment